Monitoring of dual-energy x-ray absorptiometry measurement in clinical practice.
- Détails
- Mis à jour le 24 juin 2012
Osteoporosis is a worldwide major public health problem [12]. Bone densitometry has become the “gold standard” in its diagnosis and treatment evaluation. With its advantages of high precision short scan times low radiation dose and stable calibration dual-energy x-ray absorptiometry (DXA) has been established by the World Health Organization (WHO) as the technique of reference for assessing bone mineral density (BMD) in postmenopausal women and based the definitions of osteopenia and osteoporosis on its results [3]. Recently efficient therapeutic options for treatment of osteoporosis have been developed which create possibilities of effective intervention. Therefore screening for and treatment of osteoporosis are widely practised in postmenopausal women and in people with an increased risk of osteoporosis because of underlying diseases (e.g. chronic rheumatic diseases especially when treated by corticosteroids) [4-6]. Moreover BMD measurement is needed to select patients for osteoporosis treatment as there is no proof that drugs for osteoporosis (other than hormone replacement therapy [HRT]) are beneficial in women with clinical risk factors for fractures but normal BMD values.
It has also become more and more common to perform a second DXA measurement to monitor BMD status or the effect of therapeutic intervention. When a second measurement is performed on a patient the clinician needs to distinguish between a true change in BMD and a random fluctuation relatedto variability in the measurement procedure. The reproducibility of DXA measurements is claimed to be good. Suchvariability is due to multiple causes such asdevice errors technician variability patients’movements and variation due to other unpredictable sources [7-11].
The precision error is usually expressed as the coefficient of variation (CV) [12-14] which is the ratioof the standard deviation (SD) to the meanof the measurements although several other statistics to express reproducibility exist such as the smallest detectable difference (SDD) or the least significant change (LSC).The SDD represents a cut-off thatcan be measured in an individual and is usually considered more useful than the CV in clinical practice.
Methods of bone mineral density reproducibility measurement
Precision errors are evaluated by performing repeated scans on a representative set of individuals to characterize the reproducibility of the technique. Most published studies examine the short-term precision error based on repeated measurements of each subject performed over a time period of no more than 2 weeks. Over such a short period no true change in BMD is expected.
The coefficient of variation (CV)
The CV the most commonly presented measure for BMD variability is the SD corrected for the mean of paired measurements. CV expressed as a percentage is calculated as CV (%) = (Ö((å(a-b)2)/2n))/((Ma+Mb)/2)x100 where a and b are the first and the second measurement Ma and Mb are the mean values for the two groups and n is the number of paired observations.
Reproducibility is far better for BMD measurement than for most laboratory tests. Reproducibility expressed by the CV is usually 1–2% at the spine on anteroposterior images and 2–3% at the proximal femur in individuals with normal BMD values; the difference between the two sites is ascribable to greater difficulties with repositioning and examining the femur as compared to the spine. However these data obtained under nearly experimental conditions may not apply to everyday clinical practice. Reproducibility depends heavily on quality assurance factors including tests to control the quality and performance of the machine as well as the experience of the operator. Assessment of machine performance requires daily scanning of a phantom (which may be anthropomorphic or not) followed by calculation of the in vitro coefficient of variation (CV) which serves to evaluate short-term and longterm performance and to detect drift in measurement accuracy. These in vitro data however do not necessarily reflect in vivo reproducibility which should be evaluated at each measurement centre. Measurements are obtained either three times in each of 15 patients or twice in each of 30 patients and the CV (m/r) is calculated from the mean (m) and standard deviation (r) of these repeated measurements. The CV is expressed as a percentage and depends on mean BMD values [6]. The standard deviation reflects measurement error which is a characteristic of machine performance and is independent from the value measured.
The least significant change (LSC)
For two point measurements in time a BMD change exceeding 2Ö2 times the precision error (PE) of a technique is considered a significant change (with 95% confidence): the corresponding change criterion has been termed "least significant change" or LSC. LSC = 2.8 x PE; where PE is the largest precision error of the technique used (or more easily the CV expressed in percentage). This smallest change that is considered statistically significant is also expressed in percentage [15].
The smallest detectable difference (SDD)
The measurement error can be calculated using Bland and Altman’s 95% limits of agreement method [16]. Precision expressed by this method gives an absolute and metric estimate of random measurement error also called SDD. In this case where there are two observations for each subject the standard deviation of the differences (SDdiff) estimates the within variability of the measurements. Most disagreements between measurements are expected to be between limits called ‘‘limits of agreement’’ defined as d±z(1-a/2) SDdiff where d is the mean difference between the pairs of measurements and z(1-a/2) is the 100(1-a/2)th centile of the normal distribution. The value d is an estimate of the mean systematic bias of measurement 1 to measurement 2. d is expected to be 0 because a true change in BMD is not assumed to occur during the interval between the two BMD measurements. Defining a to be 5% the limits of agreement are +1.96SDdiff and -1.96SDdiff. Thus about twice the standard deviation (SD) of the difference scores gives the 95% limits of agreement for the two measurements by the machine. A test is considered to be capable of detecting a difference in absolute units of at least the magnitude of the limits of agreement.
Clinical implications of bone mineral density reproducibility measurement
In clinical practice two absolute values (g/cm2) have to be compared rather than two percentages (T-scores). When serial measurements are obtained in a patient only changes greater than the LSC (in %) or the SDD (in g/cm2) can be ascribed to treatment effects. Smaller changes may be related to measurement error.
We studied recently the in vivo short term variability of BMD measurement by DXA in three groups of subjects with a wide range of BMD values: healthy young volunteers postmenopausal women and patients with chronic rheumatic diseases (most of them taking corticosteroids). In all studied subjects reproducibility expressed by different means was good and independent from clinical and BMD status. Thus the clinician interpreting a repeated DXA scan of a subject should be aware that a BMD change exceeding the LSC is significant in our centre arising from a BMD change of at least 3.56% at the total hip and 5.60% at the spine. Expressed as SDD a BMD change should exceed 0.02 g/cm2 at the total hip and 0.04 g/cm2 at the spine before it can be considered a significant change [17]. Indeed it has become usual to perform repeated DXA measurement: in postmenopausal women to monitor efficacy of treatment [1819] and in patients with chronic rheumatic diseases where high prevalence of bone loss has been demonstrated [20-22] especially when long term corticosteroid therapy is used. In the reports published variability is usually expressed as CV and the figures for short term variability are lower than the ones we found [7-9]. However two studies showed variability data more in line with our results. In Ravaud et al. [11] study two samples of healthy (n=70) and elderly (n=57) postmenopausal women showed a CV (%) of 0.9 and 1.8 respectively at the spine and of 0.9 and 2.3 respectively at the total hip. Eastell showed an LSC (%) of 5.4 at the lumbar spine and 8 at the total hip respectively in osteoporotic postmenopausal women [23]. It has been suggested that the varying results of reproducibility studies might be explained by the ‘‘population’’ investigated; a phantom and healthy young subjects are likely to show more favourable variability than postmenopausal women possibly in part because of easier positioning for measurement [24]. However our study failed to show better variability expressed as CV (%) in young healthy volunteers [17]. Another reason advocated was that osteoarthritis in postmenopausal women may contribute to poorer variability than found in healthy young subjects. The SDD values found in our study were comparable to the figures presented by Ravaud et al. [11]. In the first group of postmenopausal women (mean age 53 years) they describe the SDD was 0.02 (g/cm2) at the total hip and 0.02 at the lumbar spine. In the second group described women with a mean age of 80 years these figures were 0.04 and 0.04 respectively. In Lodder et al. [9] study (Ninety five women mean age 59.9 years) the SDD was 0.04 (g/cm2) at the total hip and 0.05 at the lumbar spine. The SDD values of the children studied in this study tended to be lower than the values in the postmenopausal women (table I). Using the SDD one can state that a (BMD) change larger than the figure found is a true (BMD) change in 95% of the cases. The characteristics of the Bland and Altman method thus allow direct insight into the variability of the measurement under study (figure 1).
Table 1: comparison of BMD measurement reproducibility evaluation in two studies including various groups of patients.
|
|
El Maghraoui et al. [17] |
Lodder et al. [9] |
||||||||
|
Healthy young volunteers n = 60 28.2 ± 5.5 years |
Post menopausal women n = 102 58.1 ± 7.0 years |
Chronic rheumatic diseases n= 60 47.1 ± 12.8 years |
Post menopausal women n = 95 59.9 ± 8.1 years |
Children n = 23 11.2 ± 1.3 years |
||||||
|
LS |
TH |
LS |
TH |
LS |
TH |
LS |
TH |
LS |
TH |
|
|
Mean difference : m (SD) (systematic bias) |
-0.0071 (±0.005) |
-0.0001 (±0.003) |
0.0015 (±0.004) |
0.0006 (±0.002) |
-0.0042 (±0.006) |
0.0027 (±0.004) |
-0.001 (±0.05) |
-0.0004 (±0.04) |
-0.0009 (±0.02) |
-0.003 (±0.03) |
|
SD (random measurement error) |
0.0206 |
0.0111 |
0.0180 |
0.0109 |
0.0230 |
0.0146 |
0.0238 |
0.0184 |
0.0088 |
0.0116 |
|
SDD (g/cm2) |
±0.0403 |
±0.0218 |
±0.0353 |
±0.0213 |
±0.0450 |
±0.0286 |
±0.0466 |
±0.0361 |
±0.0172 |
±0.0228 |
|
CV (%) |
1.78 |
1.05 |
2.29 |
1.58 |
1.91 |
1.24 |
1.92 |
1.59 |
0.84 |
1.19 |
|
LSC (%) |
4.94 |
2.90 |
6.35 |
4.38 |
5.29 |
3.44 |
5.43 |
4.50 |
2.38 |
3.37 |
|
Random effects ICC (95% CI) |
0.98 (0.96 - 0.98) |
0.99 (0.99 - 0.99) |
0.99 (0.98 - 0.99) |
0.99 (0.99 - 0.99) |
0.99 (0.99 - 0.99) |
0.99 (0.99 - 0.99) |
0.99 (0.98 - 0.99) |
0.99 (0.99 - 0.99) |
0.99 (0.99 – 1.00) |
0.99 (0.99 – 1.00) |
Mean difference mean of the difference between the first and the second BMD measurement; SD difference SD of the difference between the first and the second BMD measurement; SDD smallest detectable difference (g/cm2); CV coefficient of variation (%); LSC least significant change (%); ICC intraclass correlation coefficient.
(a) 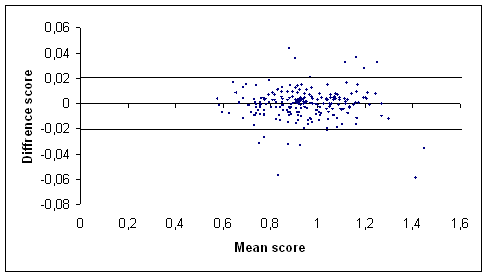
(b) 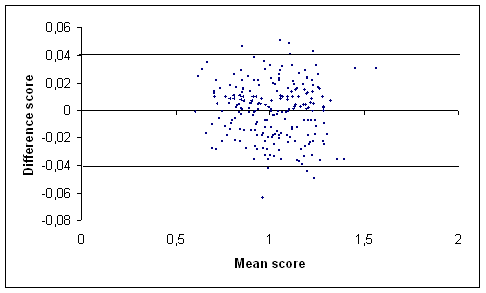
Figure 1: Graph of the difference score against the mean score of the total hip (a) and the lumbar spine (b) BMD measurements (g/cm2) in our centre [17] using Bland and Altman method. The outermost (solid) lines represent the SDD.
It has been shown that reproducibility expressed using the SDD is independent of the BMD value whereas reproducibility expressed using the CV or the derived LSC depend on the BMD value. Ravaud et al. [11] reported that using SD the values of the cut-offsare 0.024 0.030 0.020 and 0.021 g/cm2 for postmenopausal women aged £70years and 0.040 0.033 0.033 and 0.038 g/cm2 for postmenopausal osteoporotic women aged >70years at the spine femoral neck greater trochanterand total hip respectively. Using CV cut-offs varydepending on the BMD level. In postmenopausal womenaged >70years for a BMD level between 0.600 g/cm2 and 1.000 g/cm2the cut-offs derived from CV vary between 0.015g/cm2 and 0.024 g/cm20.024 g/cm2 and 0.041 g/cm20.018 g/cm2 and 0.030 g/cm20.015 g/cm2 and 0.025 g/cm2 for the spine femoral neck greater trochanter andtotal hip respectively. In postmenopausal osteoporotic women aged>70years for the same range of BMD levelcut-offs vary between 0.031 g/cm2 and 0.051 g/cm20.038 g/cm2 and 0.063 g/cm20.043 g/cm2 and 0.071 g/cm20.038 g/cm2 and 0.063 g/cm2 for the same bone sites.Consequently to express variability on a percentagebasis using CV leads to underestimate variability in patients with low BMD and to overestimate variabilityin patients with high BMD [11].Previous reports in the literatureas well as Ravaud [11] Lodder’s data [9] and our data [17] (table 1) demonstrate that absoluteprecision errors derived from SD are constant across a wide rangeof BMD values and independent of the level ofBMD. Because of therapeutic consequences the clinician should be especially careful in judging an apparent BMD change in patients with osteoporosis. Influence of age on BMD reproducibility is controversial. Previousstudies have suggested that BMD measurement errors wereindependent of age even some studies suggested that SDD may vary in extreme ages (children and elderly) probably because of age-related factors other than BMD. Howevera few data exist for reproducibility ofDXA in women over 70. Ravaud et al. [11] data as well as those of Fuleihan [12] andMaggioet al.[10] show that the measurement error is greater inolder osteoporotic subjects. Several factors such as difficulties in repositioning couldexplain the increase of measurement error in this kind of patients. Therefore the use of the SDD in the evaluation of an apparent BMD change gives a more conservative approach than the use of the CV at low BMD. Because of its independence from the BMD level and its expression in absolute units the SDD is a preferable measure for use in daily clinical practice as compared with the CV and the derived LSC.
In contrast with all previous publications about DXA reproducibility we found in our centre better results for the hip BMD variability than the lumbar spine. This is due to the fact that our study was the first to use the mean measure of the two femurs (dual femur). In this study we showed in a group of young healthy volunteers that the SDD was ±0.0218 g/cm2 when both femurs were measured whereas it was ±0.0339 g/cm2 when only one femur was measured. Thus these results enhance to encourage the use of the measurement of both hips to improve the reproducibility of DXA at this site [17].
Although the variability as expressed by the CV and especially the SDD is reassuring showing good short term variability at group level the wide range of the differences in BMD and the derived T scores indicates considerable individual differences between two consecutive BMD measurements in some patients. The range in DT scores for example indicates that in some patients the diagnosis based on the diagnostic thresholds of the WHO would change owing to the measurement variability.
In summary reproducibility of BMD measurement by DXA in different kinds of patients (postmenopausal women patients with chronic rheumatic diseases elderly…) expressed by different means is good at a group level. However the clinician must remain aware that an apparent BMD change in an individual patient may represent a precision error. At each measurement centre the SDD should be calculated from in vivo reproducibility data. In clinical practice the SDD should be used to estimate the significance of observed changes in absolute values.
Other factors influencing DXA monitoring
The first factor is the time interval between two measurements in the same patient which must be long enough to allow occurrence of a change greater than the SDD or the LSC. Therefore it depends on the expected rate of change in BMD measurement (which varies according to whether the measurement site is composed predominantly of trabecular or of cortical bone) and the reproducibility of BMD measurement at that site. Thus in clinical practice a treatment-induced BMD increase can only be detected in general after 2 years. However in patients receiving long term steroid therapy the changes in BMD may be so important that they can be detected at 1 year. Thus although the spine may not be the best site for the diagnosis of osteoporosis given the high prevalence of spinal degenerative disease it is the most sensitive site for detecting changes over time. However our study showed that measurement of both femurs (called “dual femur” in Lunar machines) increases the reproducibility at this site.
In another side the changes in BMD measurements are influenced by the ability of osteoporosis treatments to increase the BMD at the different skeletal sites. For some treatments such as teriparatide and the more potent bisphosphonates statistically significant changes in spine BMD occur on time scales of 1 to 2 years in the majority of patients although for other treatments such as raloxifene the changes are often not large enough to be statistically significant. Thus with the exception of HRT treatment dosages cannot be adjusted on the basis of BMD changes. Moreover there is no proof that repeating BMD measurements improves compliance as most patients discontinue antiresorptive medications after a few months because of administration constraints side effects cost of medications or lack of interest.
Above all BMD is used as a surrogate marker for the fracture risk yet BMD increases do not reliably reflect a reduction in the fracture risk. Although bisphosphonates raloxifene and HRT have not been compared in the same study they seem to produce comparable reductions in the risk of vertebral fractures of about 30–50% whereas BMD changes differ markedly across medications. Studies have shown that BMD gains explain only a small proportion of the vertebral fracture risk reduction: 28% with risedronate [25] 16% with alendronate [26] and 4% with raloxifene [27]. It has been suggested that the percentage of BMD change may be related to the change in the relative risk of fracture [28]. In one study a linear relationship was found between these two parameters but a 1% increase in spinal BMD was associated with an only 3% decrease in the relative risk of vertebral fracture [26]. For peripheral fractures in contrast the risk reduction is clearly related to the BMD gain [29]. Common sense indicates that a BMD increase during treatment should be preferable over a BMD decrease. However recent data showing that the fracture risk may decrease despite a reduction in BMD have been reported [30]. It has also been shown that the fracture risk was more heavily dependent on BMD at baseline than on BMD changes during treatment [31].
Conclusion
Serial BMD measurements can be used to monitor current antiresorptive treatments (bisphosphonates or raloxifene). However adequate quality-control procedures must be used [32]. Measurement error must be considered when evaluating serial assessments. A clear understanding of the interpretation of serial measurements and the statistical principles impacting upon their interpretation is necessary to determine whether a change is real and not simply random fluctuation. It is inadequate to simply use the manufacturer’s default precision
error which may underestimate the precision error in the clinical setting. Thus every centre should calculate its own precision error from in vivo reproducibility data. International societies interested in osteoporosis diagnosis and management such as the International Society for Clinical Densitometry or the International Osteoporosis Foundation should add to their guidelines at least two recommendations about DXA monitoring highlighted in this paper: the measurement of both hips improves the reproducibility at this site and DXA measurement centres should determine and use theindividual SDD. Indeed the use of the SDD is preferable to the use of the CV and LSC because of its independence from BMD level and its expression in absolute units. The exact definition and advices for the measure and use of these parameters in clinical practice should be clearly explained. It is clear that the choice of the optimum site for performing follow-up scans depends on the ratio of the BMD treatment effect to the precision of the measurements. The larger this ratio the more statistically significant the observed changes are likely to be [33]. Actually all data agree in showing that the spine is the optimum site. In clinical practice BMD measurements have to be spaced at least 2 years apart. The main goal of serial BMD measurement is to check that no further bone loss has occurred; estimation of BMD gains is the secondary objective. This should be explained to the patients many of whom expect to recover normal BMD values.
1. El Maghraoui A Koumba BA Jroundi I Achemlal L Bezza A Tazi MA. Epidemiology of hip fractures in 2002 in Rabat Morocco. Osteoporos Int 2005; 16: 597-602.
2. Bono CM Einhorn TA. Overview of osteoporosis: pathophysiology and determinants of bone strength. Eur Spine J 2003; 12 Suppl 2:S90-S96.
3. Kanis JA Johnell O Oden A Jonsson B De Laet C Dawson A. Risk of hip fracture according to the World Health Organization criteria for osteopenia and osteoporosis. Bone 2000; 27: 585-90.
4. Yeap SS Hosking DJ. Management of corticosteroid-induced osteoporosis. Rheumatology (Oxford) 2002; 41: 1088-94.
5. Maricic M Gluck O. Densitometry in glucocorticoid-induced osteoporosis. J Clin Densitom 2004; 7: 359-63.
6. El Maghraoui A. Corticosteroid induced osteoporosis. Press Med 2004; 33:1213-17.
7. Phillipov G Seaborn CJ Phillips PJ. Reproducibility of DXA: potential impact on serial measurements and misclassification of osteoporosis. Osteoporos Int 2001; 12: 49-54.
8. Nguyen TV Sambrook PN Eisman JA. Sources of variability in bone mineral density measurements: implications for study design and analysis of bone loss. J Bone Miner Res 1997; 12: 124-35.
9. Lodder MC Lems WF Ader HJ Marthinsen AE van Coeverden S.C.C.M. Lips P. et al. Reproducibility of bone mineral density measurement in daily practice. Ann Rheum Dis 2004; 63:285-9.
10. Maggio D McCloskey EV Camilli L Cenci S Cherubini A Kanis JA et al. Short-term reproducibility of proximal femur bone mineral density in the elderly. Calcif Tissue Int 1998; 63: 296-9.
11. Ravaud P Reny JL Giraudeau B Porcher R Dougados M Roux C. Individual smallest detectable difference in bone mineral density measurements. J Bone Miner Res 1999; 14: 1449-56.
12. Fuleihan GE Testa MA Angell JE Porrino N Leboff MS. Reproducibility of DXA absorptiometry: a model for bone loss estimates. J Bone Miner Res 1995; 10:1004-14.
13. Rozenberg S Vandromme J Neve J Aguilera A Muregancuro A Peretz A et al. Precision and accuracy of in vivo bone mineral measurement in rats using dual-energy X-ray absorptiometry. Osteoporos Int 1995; 5: 47-53.
14. Nguyen TV Eisman JA. Assessment of significant change in BMD: a new approach. J Bone Miner Res 2000; 15:369-72.
15. Gluer CC. Monitoring skeletal changes by radiological techniques. J Bone Miner Res 1999; 14:1952-62.
16. Bland J Altman D. Statistical methods for assessing agreement between methods of clinical measurement. Lancet 1986; i: 307-310.
17. El Maghraoui A Do Santos Zounon AA Jroundi I et al. Reproducibility of bone mineral density measurements using dual X-ray absorptiometry in daily clinical practice. Osteoporos Int 2005;16:1742-8
18. Johnson SL Petkov VI Williams MI Via PS Adler RA. Improving osteoporosis management in patients with fractures. Osteoporos Int 2005; 16: 1079-85.
19. Kanis JA Devogelaer JP Gennari C. Practical guide for the use of bone mineral measurements in the assessment of treatment of osteoporosis: a position paper of the European foundation for osteoporosis and bone disease. The Scientific Advisory Board and the Board of National Societies. Osteoporos Int 1996; 6: 256-61.
20. El Maghraoui A Borderie D Edouard R Roux C Dougados M. Osteoporosis body composition and bone turnover in ankylosing spondylitis. J Rheumatol 1999; 26:2205-9.
21. Maillefert JF Aho LS El Maghraoui A Dougados M Roux C. Changes in bone density in patients with ankylosing spondylitis: a two-year follow-up study. Osteoporos Int 2001;12: 605-9
22. El Maghraoui A. Osteoporosis and ankylosing spondyltis. Joint Bone Spine 2004; 71:573-8.
23. Eastell R. Assessment of bone density and bone loss. Osteoporos Int 1996; 6 (suppl):3-5.
24. Glüer CC Blake G LuY Blunt BA Jergas M Genank HK. Accurate assessment of precision errors: how to measure the reproducibility of bone densitometry techniques. Osteoporos Int 1995; 5: 262–70.
25. Li Z Meredith MP Hoseyni MS. A method to assess the proportion of treatment effect explained by a surrogate end-point. Statist Med 2001; 20:3175–88.
26. Cummings SR Karpf DB Harris F Genant HK Ensrud K Lacroix AZ et al. Improvement in spine bone density and reduction in risk of vertebral fractures during treatment with antiresorptive drugs. Am J Med 2002; 112:281–9.
27. Sarkar S Mitlak BH Wong M Stock JL Black DM Harper KD. Relationship between bone mineral density and incident vertebral fracture risk raloxifene therapy. J Bone Miner Res 2002; 17:1–10.
28. Wasnich RD Miller P. Antifracture efficacy of antiresorptive agents are related to changes in bone density. J Clin Endocrinol Metab 2000; 85: 231–6.
29. Hochberg MC Greenspan S Wasnich RD Miller P Thompson DE Ross PD. Changes in bone density and turnover explain the reductions in incidence of non-vertebral fractures that occur during treatement with antiresorptive agents. J Clin Endocrinol Metab 2002; 87: 1586–92.
30. Watts N Geusens P Barton IP Felsenberg D. Relationshipbetween changes in BMD and nonvertebral fracture incidenceassociated with risedronate: reduction in risk of nonvertebralfracture is not related to change in BMD. JBone Miner Res2005;20: 2097-104
31. Hochberg MC Ross PD Black D Cummings SR Genant HK Nevitt MC et al. Larger increases in bone mineral density during alendronate therapy are associated with a lower risk of new vertebral fractures in women with postmenopausal osteoporosis. Arthr Rheum 1999; 42: 1246–54.
32. Roux C Garnero P Thomas T Sabatier JP Orcel P Audran M; Comité Scientifique du GRIO. Recommendations for monitoring antiresorptive therapies in postmenopausal osteoporosis. Joint Bone Spine 2005; 72: 26-31.
33. Blake GM Fogelman I. Dual energy x-ray absorptiometry and its clinical applications. Semin Musculoskelet Radiol 2002; 6: 207-18.
